Anna Montemurro
ABSTRACT – The present
article contains a study on triangles.
Such a study shows how to
reach the solution of a triangle using an innovative method which does
not require difficult reasonings or complicated trigonometrical formulas, but
simply uses the concept of ratio between two quantities.
1. INTRODUCTION
The work begins with some observations on the triangles. They follow the
demonstrations of two new theorems. The demonstration of an innovative method
for the solution of the triangles and some examples of application.
2. OBSERVATIONS ON TRIANGLES
We want to construct one of
the innumerable triangles that has the altitude given, for example of 2 cm and
to calculate the measures of its sides. (Fig. 1).
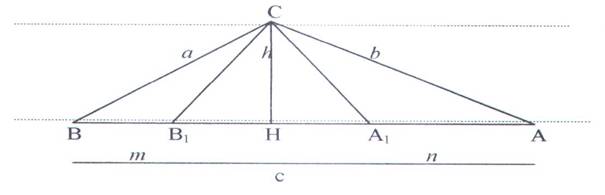
Fig. 1
We can go on as follow:
first we multiply the measure h of the altitude CH for any greater
number than the unit,, said factor k ,
then for its inverse, that is for ![]() and the products so obtained are
considered respectively as the amount of the side a and of its projection on the base that is m and as their difference, for which it results:
and the products so obtained are
considered respectively as the amount of the side a and of its projection on the base that is m and as their difference, for which it results:
![]() (1)
(1)
![]() (2)
(2)
This process finds
confirmation, multiplying member to member the (1) and the (2),we obtain the
known relation:
![]() (3)
(3)
Now, knowing the amount and the difference of two segments, to find the
measure of each of them, it is sufficient to apply the following formulas:
![]() (4)
(4)
![]() (5)
(5)
Replacing the corrrisponding values of the (1) and (2) with the (4) and (5),we obtain:
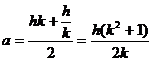 (6)
(6)
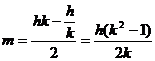 (7)
(7)
With a method similar to the previous one, choosing another major number
than the unit, said facto ![]() ,
, ![]() and
and ![]() are built and the measures b
and n are calculated.
are built and the measures b
and n are calculated.
Therefore we have:
![]() (8)
(8)
![]() (9)
(9)
![]() (10)
(10)
![]() (11)
(11)
The (6) and (7), (10) and (11)
allow to calculate the measures of the sides of one of the endless
triangles that have the altitude of 2 cm, built by us. As among the endless triangles that have the
altitude of 2 cm, there are also the right-angled triangles (in which such altitude becomes that relative
one to the hypotenuse),we ask:
what is the relation that lies![]() and
and![]() in such triangles?
in such triangles?
That is, if for example k = 6,
what value will take![]() so that such triangle is rectangle?
so that such triangle is rectangle?
From the previous observations
the theorem k (by Montemurro) and the theorem
of altitude (by Montemurro)
originate.
3.
THEOREM k (by Montemurro). In every right-angled triangled, if we have
![]() e
e ![]() then:
then:
![]() .
.
DEMONSTRATION. Given a
right-angled triangle ABC, right Ĉ, we draw the altitude relative to the
hypotenuse and a, b, c, m, n, h, are respectively the measures of the catheti, of
the hypotenuse, of the projections of the catheti on the hypotenuse and the
altitude relative to it(Fig. 2).
Fig.2
![]()
 C
C
a h b
![]() m n
m n
B H
A
![]() c
c
It is well-known that :
![]() (12)
(12)
Replacing in this last one to a, b, c, the formulas we have found
previously in the introduction, that is:
![]() (13)
(13)
![]() (14)
(14)
![]() (15)
(15)
We have:
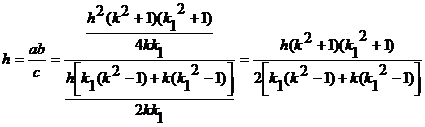 (16)
(16)
From this last one, dividing for h
≠0 both the members of the equality, we have:
![]() (17)
(17)
The (17) can be formulated easily in the following way:
![]() (18)
(18)
and, resolving as to k1
, being the discriminant of the above-said equation similar to zero, we obtain:
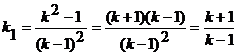 (19) c.v.d.
(19) c.v.d.
To reply to the question given in the introduction, if ![]() = 6, then:
= 6, then:
![]()
4. COROLLARY 1. One of the consequences of the theorem ![]() is that, replacing
is that, replacing ![]() in the (10) and (11) and in all the forms in
which it appears with the value
in the (10) and (11) and in all the forms in
which it appears with the value ![]() , we have all the elements of a right-angled
triangle in function of h and k :
, we have all the elements of a right-angled
triangle in function of h and k :
![]() (20)
(20)
![]() (21)
(21)
![]() (22)
(22)
![]() (23)
(23)
![]() (24)
(24)
![]() (25) where 2p indicates the perimeter
(25) where 2p indicates the perimeter
![]() (26)
(26)
![]() (27)
where ri is
inradius
(27)
where ri is
inradius
![]() (28)
(28)
Observations.
·
Relating the previous formula two by two, we can have
the other ones.
Examples.
from (20) and (22) we have: ![]() (29)
(29)
from (24) and (22) we have: ![]() (30)
(30)
from (20) and (25) we have: ![]() (31)
(31)
from (25) and (26) we have: ![]() (32)
(32)
·
Adding member to member the (1) and the (7) we obtain
the following notable ratio that is very useful in the resolution of some
problems.
![]() (33)
(33)
·
For the similarity of the right-angled
triangles BHC, AHC, … (Fig.2) there are
the following chains of equal ratios :
![]()
![]()
·
Two right-angled triangles are similar if, and only
if, they have the same value of k .
6. THEOREM OF ALTITUDE (by
Montemurro). In every triangle if we put
![]() and
and ![]() then:
then:
![]()
Note. From now on, for
facility of study, the differences ![]() e
e ![]() will be indicated respectly with
the letters e and f .
will be indicated respectly with
the letters e and f .
DEMONSTRATION. We consider any kind of
triangle ABC, with the base AB, we draw the altitude relative to it and they
are h,
e, f respectively the measures of the altitude CH, of ![]() and
and ![]() (Fig.4).
(Fig.4).
|
Fig.4 |
![]()
![]()
C
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
 e f
e f
![]() a h b
a h b
![]()
![]() B
m H
n A
B
m H
n A
![]() c
c
We want to demonstrate that:
![]() (34)
(34)
The relation (34) is
demonstrated easily because it is
given multiplying member to member the
(2) and (9) of this work.
As the product ![]() is only a number and
is only a number and ![]() expresses the area of the rectangle having for dimension
expresses the area of the rectangle having for dimension ![]() and
and ![]() , the theorem of the
altitude can be explained geometrically in the following way:
, the theorem of the
altitude can be explained geometrically in the following way:
In
each triangle the square built on one of its altitudes is equal to as many
rectangles congruent among them, each one having for dimension the measures e and f , as the product kk1 indicates .
In the right angled triangle the theorem of the altitude takes a
particular importance for the relation that is between k and k, .
7.
Corollary 2. One of the consequences of the
theorem of the altitude of a triangle is the formation of the following chain of notable reports:
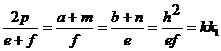 (35)
(35)
DEMONSTRATION.
With
reference to the picture 4, we consider the right angled triangles BHC and
AHC.
Applying to each of them the
theorem of Pitagora, we have that:
![]() (36)
(36)
![]() (37)
(37)
Equalizing the second members of the
(36) and (37) , we have :
![]()
That becomes :
![]()
and, for the property of to makeup:
![]() (38)
(38)
As the differences ![]() and
and ![]() have been called
respectively e and f we have:
have been called
respectively e and f we have:
![]() , from wich we obtain:
, from wich we obtain:
![]() .
(39)
.
(39)
For the equality: ![]() , we can write:
, we can write:
![]() .
.
Putting in the (39) ![]() , we obtain:
, we obtain:
![]() (40)
(40)
For the theorem of the altitude ![]() the (35) is shown.
the (35) is shown.
Note. A reader can verify
that, leaving from one of the notable ratios of (35), we can obtain some useful
formulas for the resolution of any triangle.
For example, applying the property of the
factorizing to the proportion which is obtained from the ratio ![]() , we have:
, we have:
![]() (41)
(41)
Other formulas, which we can obtain easily manipulating
those given before, are :
![]() (42)
(42)
![]() (43)
(43)
8. TRIGONOMETRIC NATURE OF k
From the relation ![]() we obtain that:
we obtain that: ![]() (44)
(44)
Putting in the (44):
![]() we have:
we have:
![]() from which we obtain:
from which we obtain:
![]() (45)
(45)
Resolving the system:
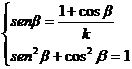
We have:
![]() (46)
(46)
![]() (47)
(47)
9.
DESCRIPTION OF THE INNOVATIVE METHOD
The innovative method for the resolution of a triangle consists in
putting always in ratio the measures given in order to calculate the value
k if we
deal with a rectangle triangle, or the values k and k1 if we deal with any kind of triangle.
Found the value k or
the values of k and k1, we
calculate the measures required using the formulas that derive from the present
work.
10. APPLICATIONS TO THE RIGHT-ANGLED
TRIANGLES
In the treatment of the
following problems we will follow the
literal indications of the picture 5.![]()
![]()
Fig.5
C
![]()

 e
f
e
f
![]() h
h
![]() a b
a b
![]()
![]() m n
m n
B H A
c
·
The perimeter and
the altitude relative to the hypotenuse of a right-angled triangle are
respectively 14,4 cm and 2,88 cm. To
calculate the measures of the sides of the triangle.
Putting the measures given in ratio and utilizing the (33), we have:
![]()
From which, resolving the equation in k , we find that ![]()
Therefore, we obtain the measures required:
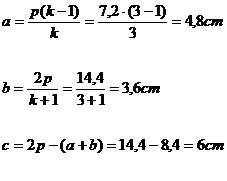
·
Is it possible to
build a right-angled triangle that has 2p=136 cm, m=45 cm ?
Solving the equation in k
which is obtained from the rapport of the measures given with the use of the
(25) and (21) and leaving the roots ![]() , we have that
, we have that ![]() . So , the answer to the question is affermative.
. So , the answer to the question is affermative.
To calculate the measures of the sides of the triangle we apply the same
formulas of the previous exercise.
·
In a right-angled
triangle we have : a =18 cm; ri =6
cm. To calculate the measures of the other sides of the triangle.
We put in ratio the measures
given, using the (20) and (27):
![]()
Therefore, we get ![]() and the measures required are
obtained respectively from the ratio of (22) and (20); (24) and (20) :
and the measures required are
obtained respectively from the ratio of (22) and (20); (24) and (20) :

·
In a right-angled
triangle we have:
a+b =
41 cm; c= 29 cm.
To calculate the measures a and b.
From the ratio :
![]()
We obtain that k = 2,5
Known the value k easily we calculate a = 21 cm and b = 20 cm, applying opportunely the
formulas that we find in this work.
·
Two right-angled
triangles are similar and have respectively:
A1 = 456,30 cm2 (
the first);
a = 31,2 cm; n = 5 cm ( the second).
To calculate the perimeter of the
first triangle and the ratio of similarity .
Note. The ratio of similarity will be indicated with the letter R.
By the ratio between a and n of the second triangle we find k=5.
As the two triangles are similar, the value k of the first of them is 5 too.
Therefore, applying opportunely the formulas obtained from the present
work, we have:
![]()
![]()
![]()
10.
APPLICATIONS TO ANY TRIANGLES
·
A triangle of area
360 cm2 is divided by the
altitude in two parts so that:
![]()
To establish if the triangle
given is a right-angled triangle or if is not. To calculate the measures of the
sides of the triangle.
We observe that, for the theorem k,
the relation ![]() is not true for k = 2,5;
therefore we conclude that the triangle given is not a right-angled triangle.
is not true for k = 2,5;
therefore we conclude that the triangle given is not a right-angled triangle.
With the inverse formula of the (42) we calculate the measure of the
altitude which is of 20 cm.
Therefore we have:
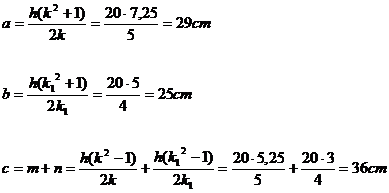
·
To calculate the
measures of the altitude and the sides of a triangle, knowing that:
a+b=150; c=120cm;
k = 6
We use the following notable
ratio:
![]()
If kk1 = 9 and k =
6, then k1 = 1,5
From: ![]()
We
find that:
![]()
Therefore:
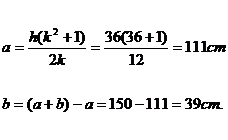
·
Two similar
triangles have respectively:
2p=216cm; h=18cm;
(the first) and,
m1=120cm, n1=36cm; (the second).
To
establish the ratio of similarity of the two triangles.
Note. The ratio of similarity of
the two triangles will be indicated with the letter R.
From the first triangle we
obtain:
![]() .
.
From:
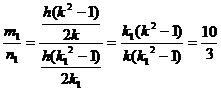 we have:
we have:
![]()
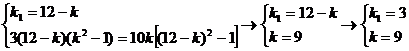
Therefore:
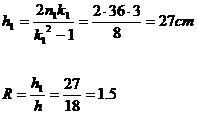
·
Two similar
triangles have respectively:
a+b=50cm; c=40cm (the first);
a1 = 92.5cm; h1 =30cm (the second).
To establish the ratio of similarity and
to calculate the measures a, b of
the first triangle.
NOTE. The ratio of similarity of the two triangles will be indicated
with the letter R..
From the first of the two triangles we obtain the value kk1
expressed from the ratio ![]()
From the second triangle we calculate the value k that is given from the ratio:
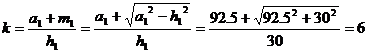
As the two triangles are similar, they have the same values k e k1 so, replacing in kk 1= 9 the value k=6
we have that k1=1,5
![]()
So we have that:
![]()
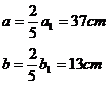
·
To resolve a
triangle ABC, with base AB, knowing that:
a+ c
=77cm; b=13cm; k=6
We calculate, as usual, the
ratio among the measures given ![]() , and we put in it :
, and we put in it :
![]()
![]() ;
; ![]() ,
,
that is:
![]() (we remember that k stands for 6).
(we remember that k stands for 6).
Resolving the equation which
is obtained from the previous relation and ruling out the root <1, we
obtained that k1 = 1.5.
Therefore,
we have:
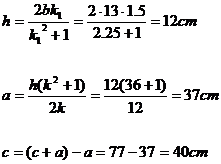
·
To resolve an obtuse-angled
triangle ABC, with base AB, knowing that :
a+c=112cm; b=104cm;
k =13/6
C
![]()
h
a b
![]()
![]()
![]() H
H ![]()
m
B c
A
![]() n
n
In this case, as the triangle given is obtuse-angled, the foot of the
altitude falls on the prolongation of the side AB; therefore, for definition,
the value k given refers to the ratio ![]() .
.
We utilize the data of the problem finding, as usual, the ratio among
the measures known, that is:
![]() (1).
(1).
Putting
in the (1) :
![]()
and, resolving the equation
that we obtain, that is:
![]() (we remember that
(we remember that ![]() ), we establish
), we establish
The value k1=3 , excluding the root equal to 9 which refers to the acute minor angle.
So we calculate the measures
required:
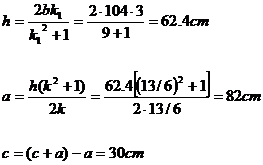
Observation. When the size of the obtuse
angle of a triangle is given , to calculate the value k ,first it is necessary to find the supplementary angle of that
one given and, then, to apply the formula ![]() .
.
11.
Conclusion
We
can resolve a triangle, that is we can calculate all its elements: sides and
angles, following the tracking models
reported here on end and already indicated in the 9. point in the description
of the innovative method.
“We
calculate the ratio among the
measures known at the end to find the values k and k1
and we apply opportunately the formulas which flow from this work”.